

Most ebook files are in PDF format, so you can easily read them using various software such as Foxit Reader or directly on the Google Chrome browser.
Some ebook files are released by publishers in other formats such as .awz, .mobi, .epub, .fb2, etc. You may need to install specific software to read these formats on mobile/PC, such as Calibre.
Please read the tutorial at this link: https://ebookbell.com/faq
We offer FREE conversion to the popular formats you request; however, this may take some time. Therefore, right after payment, please email us, and we will try to provide the service as quickly as possible.
For some exceptional file formats or broken links (if any), please refrain from opening any disputes. Instead, email us first, and we will try to assist within a maximum of 6 hours.
EbookBell Team
4.4
102 reviewsPhysical Specifications
The booklet measures 6 x 9 inches, making it portable and convenient to carry around. It consists of 30 pages filled with valuable insights, explanations, and examples that aid in comprehending the intricacies of integration.
Key Elements Covered
Infinite Integrals: This section delves into the concept of integrating functions over an infinite range, exploring techniques such as substitution, integration by parts, and trigonometric substitutions.
Preparing to Integrate: Here, readers are introduced to important prerequisites for effective integration, including simplification of expressions, use of algebraic techniques, and identifying patterns.
Differential Equations: This segment focuses on the connection between integration and solving differential equations, enabling readers to grasp the fundamental relationship between these two mathematical concepts.
Definite Integrals: The booklet provides a comprehensive overview of definite integrals, including their properties, evaluation methods, and applications in calculating areas, volumes, and averages.
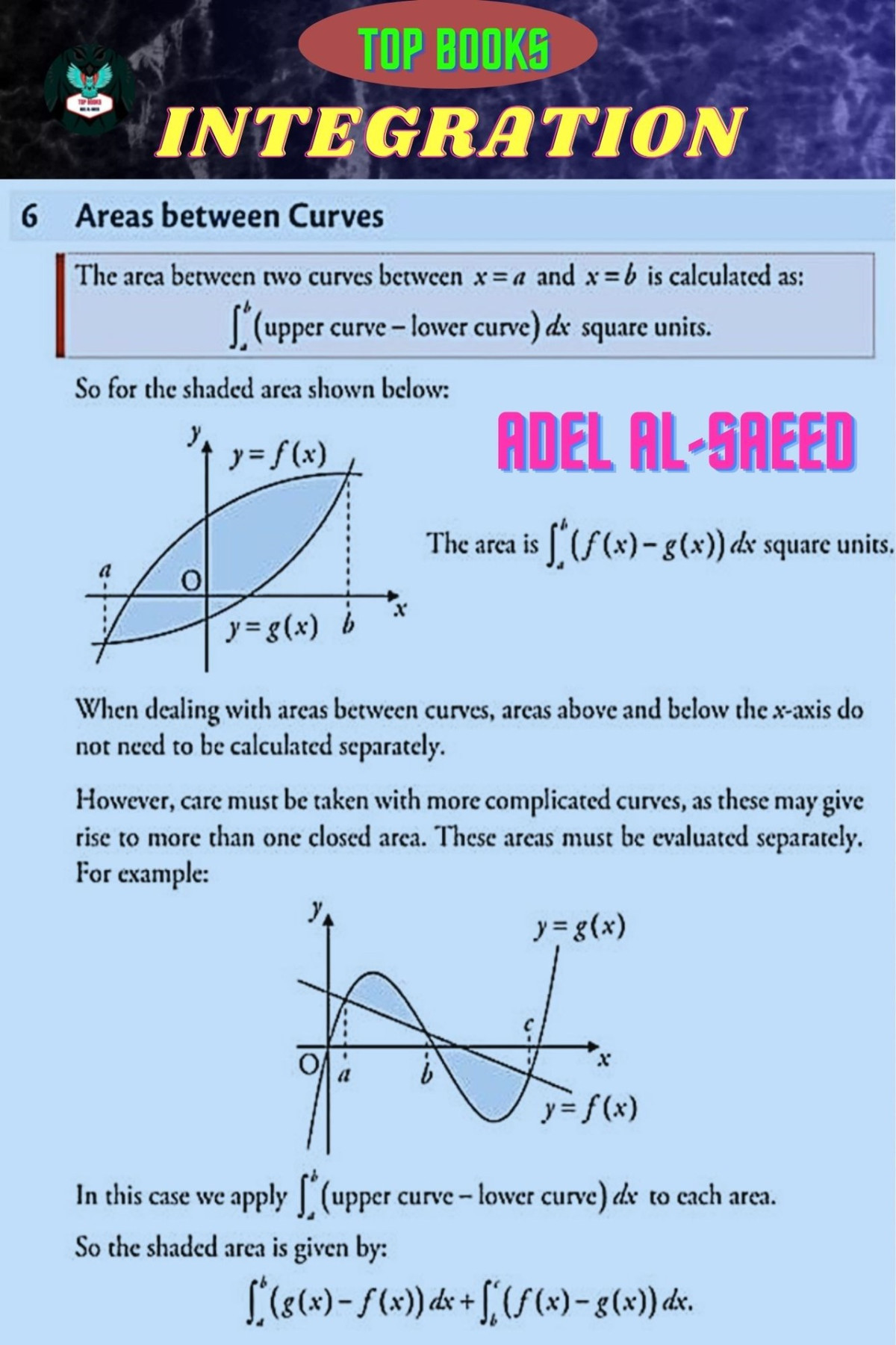
Geometric Interpretation of Integration: This section explores the geometric interpretation of integration, emphasizing the connection between integrals and the calculation of areas between curves.
Areas Between Curves: Readers will gain insights into how to calculate the areas enclosed by curves using integration techniques, with detailed explanations and examples.
Integrating Along the Y-Axis: This portion focuses on integrating functions with respect to the y-axis, offering step-by-step
…